An anonymous Persian work on ornamental geometry, On interlocks of similar or complementary figures, appears to be compiled by a scribe at a series of meetings between mathematicians and artisans. This unusual document, which contains 68 geometric constructions mostly with verbal explanations, can be dated by internal evidence to ca. 1300. Its provenance was likely Tabriz, the capital city of the Ilkhanids, which was the scene of huge construction campaigns under the sponsorship of Gazan Khan and his vizier Rashid al-Din at the turn of the fourteenth century.

Some of those constructions display the highest advancements attained by Muslim mathematicians thus represent the intimate link between theory and praxis that created the intriguing and awe-inspiring ornamental patterns. For instance, three of these are in fact the solutions to problems that require cubic equations. In those times mathematicians solved the cubic equations by means of conic sections; but such solutions were only for demonstration purposes with no practical application. These three constructions in Interlocks of Figures, which records the collaboration of mathematicians and artisans, are the cases of "moving geometry," that is to say, mechanical procedures that are equivalent to the solutions for cubic equations.

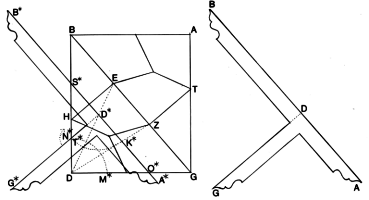
Constructions 37 (left) and 42 (right) of Interlocks of Figures

The first construction is about "a right-angled triangle such that if [a length equal to the shortest side] is cut from the hypotenuse of the triangle towards the shortest side and a perpendicular is erected at the point of cutting, it cuts off the intermediate side at a point where [the distance] from it to the right angle is equal to the perpendicular itself." The solution is achieved by trial-and-error, i.e., by moving a straightedge around a pivotal point until the required position is reached. It actually corresponds to solving the cubic equation x³ + 2x² - 2x - 2 = 0.

The second construction concerns again the same right triangle; but in this case joining two such triangles facing opposite directions completes the rectangular repeat unit of the ornamental pattern in question. This time the moving instrument was a prototype of the T-square. It revolves around the center of a circle so that the solution is achieved by the intersection of two implied hyperbolas, which is equivalent to equation x³ - 3x² - x + 1 = 0. We are told that the T-square, which appears to be introduced at that particular meeting, was meant to facilitate drawing patterns that involve conic sections. After the invention of this simple drafting instrument, we can interpret by hindsight that transmission of knowledge by way of architectural drawings-mostly ground plans based on square grids-gained impetus.

The third construction consists of four right-angled triangles rotating around a central square so as to form an ornamental pattern. The special property of this triangle is that "the altitude plus the shortest side is equal to the hypotenuse." Omar Khayyam, the celebrated poet-mathematician, had written a treatise on this triangle and offered the solution of equation x³ - 20x² + 20x - 2000 = 0 by means of conic sections. The solution in Interlocks of Figures was achieved again with the aid of the T-square. In this case, its sliding movement translates the solution of equation x³ - 4x² + 6x - 2 = 0 into the intersection of a circle and a parabola, by way of focus-directrix property. The triangle that Omar Khayyam had discovered has other properties; it embodies what the Greeks called "the musical proportion." A mathematical analysis of the North Dome Chamber (1088-89) of the Great Mosque of Isfahan reveals that its proportions were generated wholly by Omar Khayyam's triangle, thus exemplifying the active involvement of prominent mathematicians in the great accomplishments of Islamic architecture.

ABOUT THE AUTHOR
Associate Professor Alpay Özdural is currently teaching design and history of architecture courses at Eastern Mediterranean University, Faculty of Architecture in North Cyprus. He is by profession an architect specialized in restoration and preservation of historic monuments and sites. His other fields of interest are architectural photogrammetry, history of mathematics, and historical metrology. For the last ten years he has concentrated his efforts on muqarnas, a type of three-dimensional geometric decoration peculiar to Islamic architecture, and on the collaboration of mathematicians and artisans in the medieval Islamic world. He has published articles in such journals as Historia Mathematica, Technology and Culture, Muqarnas and the Journal of the Society of Architectural Historians.