We say a number is flat if it can be written as a non-trivial power of 2 times an odd squarefree number. The power is the ``exponent" and the number of odd primes the ``length". Let
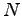
be flat and 4-perfect with exponent
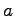
and length
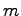
. If
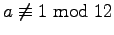
, then
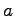
is even. If
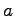
is even and
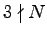
then
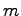
is also even. If
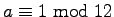
then
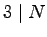
and
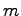
is even. If
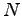
is flat and 3-perfect and
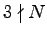
, then if
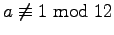
,
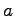
is even. If
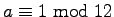
then
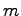
is odd. If
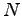
is flat and 3 or 4-perfect then it is divisible by at least one Mersenne prime, but not all odd prime divisors are Mersenne. We also give some conditions for the divisibility by 3 of an arbitrary even 4-perfect number.
Received June 30 2009;
revised versions received October 12 2009; January 7 2010.
Published in Journal of Integer Sequences, January 8 2010.

