JIPAM
| The Stability of Some Linear Functional Equations | |||||||
| Authors: | Belaid Bouikhalene, | ||||||
| Keywords: | Linear functional equations, Stability, Superstability. | ||||||
| Date Received: | 14/01/04 | ||||||
| Date Accepted: | 25/04/04 | ||||||
| Subject Codes: |
39B72. |
||||||
| Editors: | Kazimierz Nikodem, | ||||||
| Abstract: |
In this note, we deal with the Baker's superstability for the following linear functional equations
where | ||||||
http://jipam.vu.edu.au
The URL for this article is:
http://jipam.vu.edu.au/article.php?sid=381
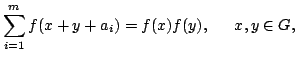
![$displaystyle sum_{i=1}^{m} [f(x+y+a_{i})+f(x-y-a_{i})]=2f(x)f(y),quadx,yin G,$](images/012_04_JIPAM/img2.gif)