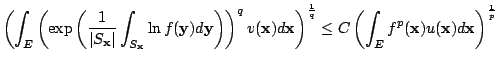JIPAM

Weighted Geometric Mean Inequalities Over Cones in $R^N$  
 
  Authors: Babita Gupta, Pankaj Jain, Lars-Erik Persson, Anna Wedestig,  
  Keywords: Inequalities, Multidimensional inequalities, Geometric mean inequalities, Hardy type inequalities, Cones in $R^{N}$, Sharp constant.  
  Date Received: 07/11/02  
  Date Accepted: 20/03/03  
  Subject Codes:

26D15,26D07.

  
  Editors: Bohumir Opic,  
 
  Abstract:

Let $ 0pleq qinfty .$ Let $ A$ be a measurable subset of the unit sphere in $ mathbb{R}^{N},$ let $ E=left{ mathbf{x}inmathbb{R}^{N}: mathbf{x}=ssigma ,0leq sinfty ,sigma in Aright} $ be a cone in $ mathbb{R}^{N}$ and let $ S_{mathbf{x}}$ be the part of $ E$ with 'radius' $ leq leftvertmathbf{x}rightvert .$ A characterization of the weights $ u$ and $ v $ on $ E$ is given such that the inequality

$displaystyle left( int_{E}left( exp left( frac{1}{leftvert S_{mathbf{......left( int_{E}f^{p}( mathbf{x})u(mathbf{x})dmathbf{x}right) ^{frac{1}{p}}$    
holds for all $ fgeq 0$ and some positive and finite constant $ C.$ The inequality is obtained as a limiting case of a corresponding new Hardy type inequality. Also the corresponding companion inequalities are proved and the sharpness of the constant $ C$ is discussed.
;


This article was printed from JIPAM
http://jipam.vu.edu.au

The URL for this article is:
http://jipam.vu.edu.au/article.php?sid=309